Problem statement
Given an integer array arr, and an integer target, return the number of tuples i, j, k such that i < j < k and arr[i] + arr[j] + arr[k] == target.
As the answer can be very large, return it modulo 10^9 + 7.
Example 1
Input: arr = [1,1,2,2,3,3,4,4,5,5], target = 8
Output: 20
Explanation:
Enumerating by the values (arr[i], arr[j], arr[k]):
(1, 2, 5) occurs 8 times;
(1, 3, 4) occurs 8 times;
(2, 2, 4) occurs 2 times;
(2, 3, 3) occurs 2 times.
Example 2
Input: arr = [1,1,2,2,2,2], target = 5
Output: 12
Explanation:
arr[i] = 1, arr[j] = arr[k] = 2 occurs 12 times:
We choose one 1 from [1,1] in 2 ways,
and two 2s from [2,2,2,2] in 6 ways.
Constraints
3 <= arr.length <= 3000.0 <= arr[i] <= 100.0 <= target <= 300.
Solution 1: Bruteforce
Find all tuples that satisfy the target sum.
Code
#include <vector>
#include <iostream>
using namespace std;
int threeSumMulti(vector<int>& arr, int target) {
const int MOD = 1000000007;
int count = 0;
for (int i = 0; i < arr.size() - 2; i++) {
for (int j = i + 1; j < arr.size() - 1; j++) {
for (int k = j + 1; k < arr.size(); k++) {
if (arr[i] + arr[j] + arr[k] == target) {
count += 1 % MOD;
}
}
}
}
return count;
}
int main() {
vector<int> arr{1,1,2,2,3,3,4,4,5,5};
cout << threeSumMulti(arr, 8) << endl;
arr = {1,1,2,2,2,2};
cout << threeSumMulti(arr, 5) << endl;
}
Output:
20
12
Complexity
- Runtime:
O(N^3), whereN = arr.length. - Extra space:
O(1).
Solution 2: Store one of the values in the tuples
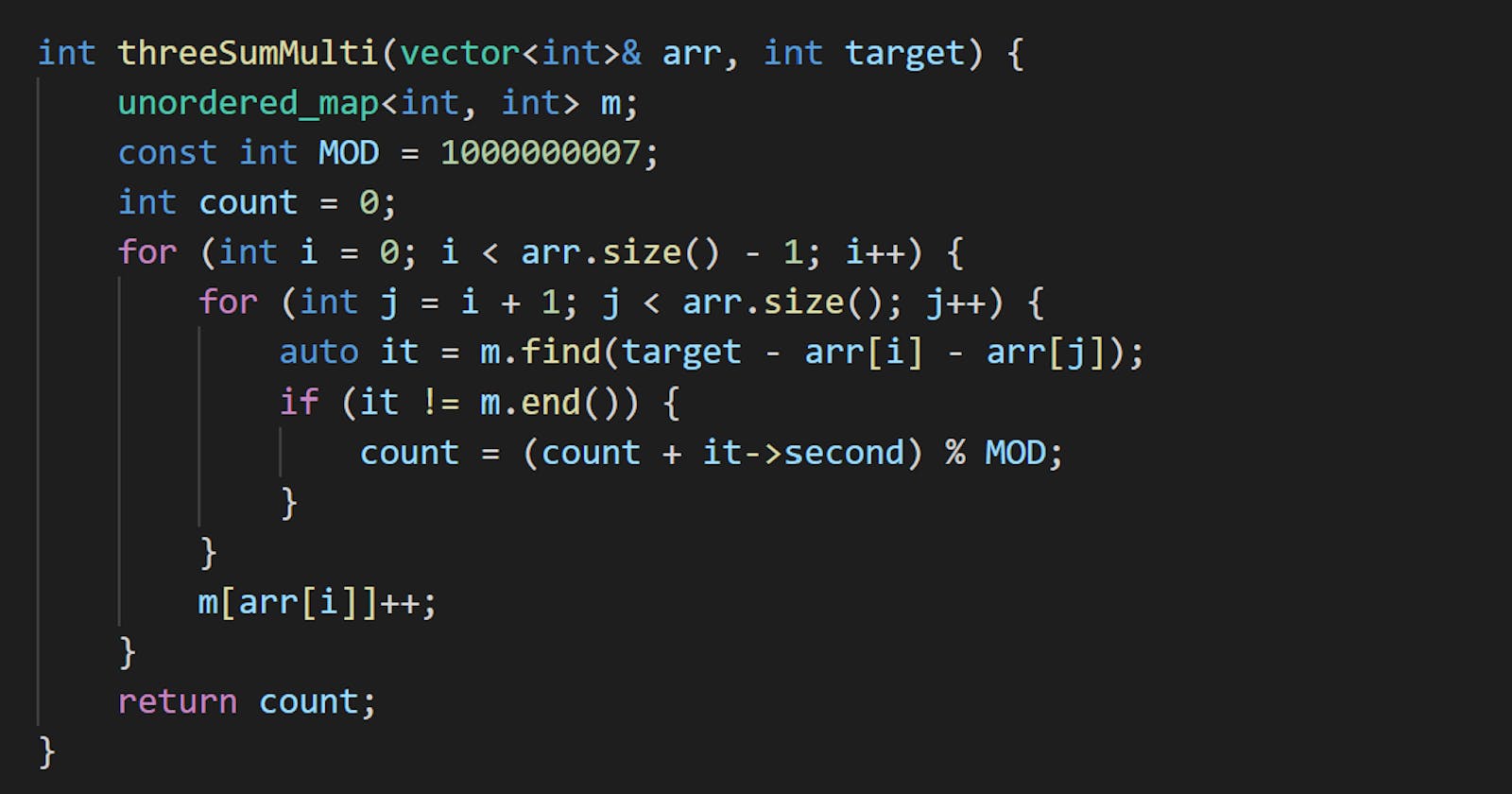
Rewrite the condition arr[i] + arr[j] + arr[k] == target as arr[i] == target - arr[j] - arr[k], then you can reduce one of the for loops by using a map to store the count of the values arr[i] that have been visited.
Code
#include <vector>
#include <iostream>
#include <unordered_map>
using namespace std;
int threeSumMulti(vector<int>& arr, int target) {
unordered_map<int, int> m;
const int MOD = 1000000007;
int count = 0;
for (int i = 0; i < arr.size() - 1; i++) {
for (int j = i + 1; j < arr.size(); j++) {
auto it = m.find(target - arr[i] - arr[j]);
if (it != m.end()) {
count = (count + it->second) % MOD;
}
}
m[arr[i]]++;
}
return count;
}
int main() {
vector<int> arr{1,1,2,2,3,3,4,4,5,5};
cout << threeSumMulti(arr, 8) << endl;
arr = {1,1,2,2,2,2};
cout << threeSumMulti(arr, 5) << endl;
}
Output:
20
12
Complexity
- Runtime:
O(N^2), whereN = arr.length. - Extra space:
O(N).